OK, there is apparently a LOT of misinformation floating around on how to calculate a loan. The most common misconception is to take $ 10,000 and multiply it by 18% to get Interest of $ 1,800. But this is highly incorrect.
The words Interest and Finance Charges are used interchangeably by most people in the financial industry.
The standard Loan Calculation goes like this:
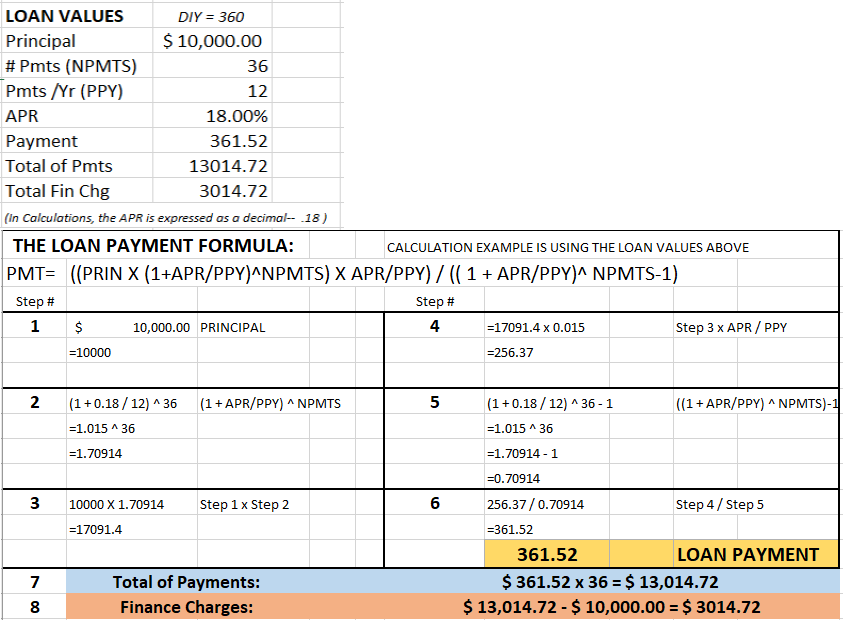
The reason why it isn’t as simple as Principal x APR is that a Loan is Amortized over a period of time, and paid in regular installments, meaning that the original Principal isn’t loaned out for the entire time, it is paid back gradually. Since the lender collects some of the Principal with each payment, the interest declines to reflect only the amount of interest due on the current Principal Balance. The formula above is the best way to achieve a payment that reflects a Declining Interest Amortization payment.
The following image is the Estimated Amortization for this loan. Note how the interest on each payment Declines to reflect the interest owed for the Principal Balance for that payment date.

The above Estimated Payment Schedule uses a standardized 30 days for each period to arrive at the Interest Due for a payment. The reality is, that if you pay on the exact due dates, then the days passed between payment dates is not 30 each time. In fact, of these 36 payments, only 12 are 30 day periods, and 21 are 31 day periods. There are 2 periods of 28, and 1 of 29 days.
Since the rules allow the lender to calculate the interest using the exact days, the interest being paid will vary on 24 of the 36 payments, 21 of them being higher than estimated. The calculation for the interest due for any given payment is: Principal x (APR/ DIY) = Interest per day. IPD x Days = Interest Due. “Days” refers to the number of days since the last payment.
Let’s look at the Estimate again, this time with Actual Days being used on the right side to show the payment breakdowns.

Adding the Interest from the Actual Days together, the consumer will pay $3,077.74 , not the Estimated $3014.72. And, due to paying 63.02 extra in Interest, there is now 63.02 Principal left owing after the last payment.
Keep in mind, too, that when the consumer pays on a date that is not on this schedule, the amount of Interest Due changes to that payment date, and that can change the rest of the schedule. For example, let’s say this customer pays the first payment 10 days late. The Interest Per Day is 5.00 at that point, so the Interest Due becomes 200.00 instead of 150.00. Paying just the normal payment amount allocates that amount to Interest, and the principal allocation drops by 50.00, since Principal Allocation is simply Amount Paid – Interest Due.
Since the Principal balance is higher that the original estimate for Payment # 2, the interest due rises, so the allocation to principal for payment 2 is lower, making the principal balance for Payment #3 higher than expected.
That 50.00 extra interest on payment #1 costs the consumer 52.65 total over the life of the loan, even if the rest of the payments are made on time. This person now has a final principal owed of $65.67 instead of $63.02.
I hope this clears up the questions about the Interest Calculations. If not, drop a comment, and I’ll be glad to see if I can help.
1 comment